How to factor a 5-term polynomial (rational zero theorem & synthetic division)
Vložit
- čas přidán 16. 03. 2024
- Algebra tutorial on factoring a 5-term polynomial x^4-4x^3+2x-11x+12 using the rational zero theorem (aka the rational root theorem) and the synthetic division. Factoring polynomials is crucial for solving polynomial equations for your algebra and precalculus classes. Check out different methods of factoring this 5-term polynomial below.
By rational zero theorem: • How to factor a 5-term...
By grouping: • How to factor a 5-term...
The double-cross method: • How to factor a 5-term...
Shop my math t-shirts & hoodies on Amazon: 👉 amzn.to/3qBeuw6
My blue jacket: 👉 amzn.to/3qBeuw6
-----------------------------
I help students master the basics of math. You can show your support and help me create even better content by becoming a patron on Patreon 👉 / blackpenredpen . Every bit of support means the world to me and motivates me to keep bringing you the best math lessons! Thank you!
-----------------------------
#math #algebra #factoring #polynomials #tutorials



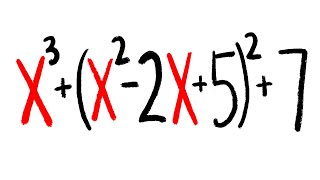





By grouping: czcams.com/video/55ufNfFofzY/video.html
The double-cross method: czcams.com/video/B8dCd6PkHMY/video.html
Instead of plugging in 1 and 4 and other numbers into the polynomial (and have to calculate sums of large powers like 4^3), just do synthetic division with each possible rational zero until you find one that gives remainder zero. You use synthetic division to factor, use it to test zeros also.
You know what! I just realized that I have never thought about that. 😆 Thanks!
Yeah! I like to check +-1 by counting the coefficients and then checking everything else via synthetic division
Synthetic is a lifesaver.
Thank's for this method! with this one i am able to factor any polynumial term!
Thank you sir ❤
we were recently dealing with partial fraction case 1 and 2 with 5 term polynomials in the last exam and it was this easy to factor out???🤯🤯🤯
Maybe the quintic solution lies in something like this. Knowing that every silhouette of a non-morphic shape has a constant K that can be clearly identified at an angle of 45° if the side is demonstrated with such a constant.Just like a square that measures 4 sides.
Then another square is made inside with the coordinates 1.25 and 3.75 to the left side height. Then complete the halves. Now divide the side of the square that is twice as large as the side of the smaller square. Each coordinate will now become a number such that than (X.Y). Now you will divide by 4 by the 4 coordinates and subtract again by 4. You will obtain a linear figure that will reveal the square constant in real numbers such that every number will end up in N,n64. The 45° angle of a circle such that the side is divided by ✓2=a number that somehow satisfies the shape's silhouette constant. In this case the 4x4 square. :) oops, I think there's something interesting here, don't you? Once the 45° angle is such that in the shape or outside it satisfies the shape constant of the silhouette in an explicit and transformable way, the quintic root can perhaps be extracted, if that can be done be proven.
Don't waste your time on this. It is provable by Galois theory that a _general_ algebraic solution of quintic equations in radicals cannot exist. This does not mean that no quintic equation can be solved algebraically. In fact, a polynomial equation is solvable by radicals if and only if its Galois group is a solvable group. There are systematic ways, however, to deal with quintic equations which involve the use of elliptic functions (Jacobi theta functions) or generalized hypergeometric functions.
after the first synthetic division, can i stop there? or do i need to keep going to 2nd synthetic division?
At the beginning of the video, you said "divide everybody by 1. it doesn't matter." What if you have a leading coefficient that is not a one? say, 5x^4 + 16x^3 + 15x^2 + 8x + 16?
I remember when my pre calc was teaching us p/q. Hated every second of guessing random zeros over and over again. Me and my friends just ended up calling large polynomials "Sysiphus equations" because honestly I rather roll a giant rock up a super high mountain.
Hey, so I found your video where you talk about 2=0 post in reddit. So i want to ask similar question.
-1 = (-1) (-1) (-1) = (-1)^3 = (-1)^(6/2) = √(-1)^6 = 1
Where's the fault in this case? I can't clearly explain the fault
Sqrt(-1)=i
i^6=-1
@@harry_dum7721 cmiiw but aren't we supposed to do the exponential first instead of the root?
@@fadillahaguma2744 Not if the base is negative. Taking a root only gives you the principal root back, so it's not the same as the inverse of exponentiation unless the base is in the same domain as the principal root.
@@phiefer3 so it's not the same case with √x², where the result are always positive (absolute value) because we do the exponent first?
@@fadillahaguma2744That's exactly what you did, you took the absolute value of a negative number, and expected it to still be equal to the original, that IS the fault.
√x² is NOT the same as (√x)² unless we restrict x to non-negative values. The latter will always return x, while the former will return |x|. You have to be mindful when manipulating exponents that you're not changing the domain due to principal roots.
Is math just applied philosophy? 5:18 "Sometimes, of course, you have to keep trying for a few times... And sometimes... None of this might work"
😆
In this video as well as in two other recent videos you discuss three methods for factoring a quartic polynomial:
1. Rational zero theorem and synthetic division
2. Factoring by grouping
3. The double-cross method
However, there is also a fourth method which does not rely on trial and error (although this can often be used to advantage). This method is based on Ferrari's method for solving quartic equations and works by converting the quartic polynomial into a difference of two squares which can then be factored into two quadratics. To see how this works, let's use this method to factor your quartic polynomial
x⁴ − 4x³ + 2x² − 11x + 12
The first step is to complete the square with respect to the quartic and cubic terms. Since x⁴ = (x²)² is the square of x² and since 4x³ = 2·(x²)·(2x) is twice the product of x² and 2x we have (x² − 2x)² = (x²)² − 2·(x²)·(2x) + (2x)² = x⁴ − 4x³ + 4x² and therefore x⁴ − 4x³ = (x² − 2x)² − 4x² so we can rewrite our quartic polynomial as
(x² − 2x)² − 4x² + 2x² − 11x + 12
which gives
(x² − 2x)² − 2x² − 11x + 12
Since the aim is to convert this into a difference of two squares, we now rewrite this as a difference of two terms by leaving the first term as it is and grouping the remaining quadratic, linear and constant terms so we get
(x² − 2x)² − (2x² + 11x − 12)
Here the first term (x² − 2x)² is already a square, but the second term (2x² + 11x − 12) is not. A quadratic polynomial ax² + bx + c can be written as the square of a linear polynomial if and only if this quadratic polynomial has two identical zeros. This is the case if and only if the discriminant b² − 4ac of this quadratic polynomial is equal to zero. The discriminant of 2x² + 11x − 12 is 11² − 4·2·(−12) = 121 + 96 = 217 so evidently 2x² + 11x − 12 is not the square of a linear polynomial in x.
Since the difference of the two terms (x² − 2x)² and (2x² + 11x − 12) remains the same if we add the same quantity to each of these two terms, we can add anything we want to the second term in order to make this into a square, provided that we add the same quantity to the first term. However, since the first term already is a square, we must ensure that this term will _remain_ a square if we add something to both terms in order for the second term to _become_ a square. How can we do that?
Well, if we take any number λ and add 2λ(x² − 2x) + λ² to both terms, then the first term will become (x² − 2x)² + 2λ(x² − 2x) + λ² = (x² − 2x + λ)² and will therefore remain a square. So, adding 2λ(x² − 2x) + λ² = 2λx² − 4λx + λ² to both terms of our quartic we get
(x² − 2x + λ)² − ((2λ + 2)x² − (4λ − 11)x + (λ² − 12))
The first term (x² − 2x + λ)² is a square regardless of the value of λ, so we are free to choose λ in such a way that the second term ((2λ + 2)x² − (4λ − 11)x + (λ² − 12)) will also become a square. The second term is a quadratic polynomial ax² + bx + c with a = 2λ + 2, b = −(4λ − 11), c = λ² − 12 and this will therefore be the square of a linear polynomial in x if its discriminant b² − 4ac is zero, that is, if λ satisfies
(4λ − 11)² − 4(2λ + 2)(λ² − 12) = 0
This is a cubic equation in λ, but we do _not_ have to solve this equation formally if our quartic polynomial happens to have a factorization into two quadratics with _integer_ coefficients.
Clearly, if our quartic has a factorization into two quadratics with integer coefficients then the second term ((2λ + 2)x² − (4λ − 11)x + (λ² − 12)) can be the square of a linear polynomial with an integer coefficient of x, so the coefficient 2λ + 2 of x² must then be the square of an integer. Also, the constant term λ² − 12 must then be the square of an integer multiple of ¹⁄₂.
So, for a potential integer factorization we only need to check values of λ that will make 2λ + 2 equal to 1, 4, 9 ... and see if λ² − 12 is the square of an integer multiple of ¹⁄₂. Now, 2λ + 2 = 1 implies λ = −½ and 2λ + 2 = 4 implies λ = 1 but both these values of λ imply λ² − 12 < 0 which means that the constant term would not be the square of a real number, much less the square of an integer multiple of ¹⁄₂. But the next possibility 2λ + 2 = 9 implies λ = ⁷⁄₂ and indeed we then have λ² − 12 = (⁷⁄₂)² − 12 = ⁴⁹⁄₄ − ⁴⁸⁄₄ = ¹⁄₄ = (¹⁄₂)² as required. With λ = ⁷⁄₂ our polynomial
(x² − 2x + λ)² − ((2λ + 2)x² − (4λ − 11)x + (λ² − 12))
becomes
(x² − 2x + ⁷⁄₂)² − (9x² − 3x + ¹⁄₄)
which is
(x² − 2x + ⁷⁄₂)² − (3x − ¹⁄₂)²
and we have converted our quartic into a difference of two squares. Using the difference of two squares identity a² − b² = (a − b)(a + b) this can be written as
(x² − 2x + ⁷⁄₂ − 3x + ¹⁄₂)(x² − 2x + ⁷⁄₂ + 3x − ¹⁄₂)
which is
(x² − 5x + 4)(x² + x + 3)
and this completes the factorization of x⁴ − 4x³ + 2x² − 11x + 12 into two quadratics with integer coefficients. Of course the first of these quadratic factors can in turn be factored as (x − 1)(x − 4) while the second of these quadratic factors cannot be factored over the integers since its discriminant is not the square of an integer. In fact x² + x + 3 cannot even be factored over the reals since the discriminant of this quadratic is negative.
Wow!! This is amazing. Thanks for sharing!
x^4-4x^3+2x^2-11x+12
(x^4-4x^3) - (-2x^2 + 11x -12)
(x^4-4x^3+4x^2) - (2x^2 + 11x -12)
(x^2-2x)^2 - (2x^2 + 11x -12)
(x^2 - 2x + y/2)^2 - ((y+2)x^2 + (-2y+11)x + y^2/4 - 12)
4*(y^2/4 - 12)*(y+2) - (-2y+11)^2 = 0
(y^2-48)(y+2) - (2y-11)^2 = 0
y^3+2y^2-48y-96 - (4y^2-44y + 121) = 0
y^3+2y^2-48y-96 - 4y^2 + 44y -121 = 0
y^3 - 2y^2 - 4y - 217 = 0
y^3 - 343-2y^2-4y+126 = 0
y^3 - 343 - 2(y^2-49) - 4y + 28 =0
y^3 - 343 - 2(y^2-49) - 4(y-7) =0
(y-7)(y^2+7y+49) - (y-7)(2y+14)-4(y-7) =0
(y-7)(y^2+5y+31) = 0
(x^2 - 2x + y/2)^2 - ((y+2)x^2 + (-2y+11)x + y^2/4 - 12)
(x^2 - 2x + 7/2)^2 - (9x^2 -3x + 1/4)
(x^2 - 2x + 7/2)^2 - (3x-1/2)^2
((x^2 - 2x + 7/2) - (3x-1/2))((x^2 - 2x + 7/2) + (3x-1/2))
(x^2 - 5x + 4)(x^2 + x + 3)