3-Body Problem Equations Derived, Part 2: Rotating Frame | Topic 3

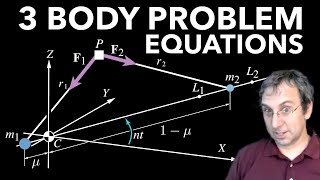
The usual rotating frame equations of motion for a spacecraft in the Circular Restricted Three Body Problem (CR3BP) are derived. This model describes the motion of a body moving in the gravitational field of two primaries that are orbiting in a circle about their common center of mass, with trajectories such as Lagrange points, halo orbits, Lyapunov planar orbits, quasi-periodic orbits, quasi-halos, low-energy trajectories, etc.
• The two primaries could be the Earth and Moon, the Sun and Earth, the Sun and Jupiter, etc.
• The equations of motion of derived in the inertial frame centered at the primaries' center of mass.
• The equations have been non-dimensionalized
• We view Newton's equations, but in a rotating frame, using rotating frame coordinates, which has the advantage of giving us time-independent (autonomous) ordinary differential equations, which allows us to use many techniques from dynamical systems theory
• The main parameter emerging is the mass parameter μ , which is the ratio of the mass of the smaller primary compared to their sum, for example for the Earth-Moon system, it is μ=0.01215
• The mass parameter μ is the only factor determining the type of motion possible for the spacecraft. It is analogous to the Reynold's number Re in fluid mechanics, as it determines the onset of new types of behavior.
▶️ Next: 3-Body Problem Lagrangian Equations and Effective Potential Energy
• 3-Body Problem Lagrang...
▶️ Previous: 3-Body Problem Equations Derived, Part 1: Inertial Frame and Nondmensionalizing
• 3-Body Problem Equatio...
▶️ In Case you Missed It: 3-Body Problem Introduction
• Three Body Problem Int...
► FREE Book on the Patched 3-Body Method:
Dynamical Systems, the Three-Body Problem and Space Mission Design. Koon, Lo, Marsden, Ross (2011)
shaneross.com/b...
We are Chapter 2.
► PDF Lecture Notes (Lecture 2 for this video)
is.gd/3BodyNotes
► Dr. Shane Ross is an Aerospace Engineering Professor at Virginia Tech. He has a Ph.D. from Caltech (California Institute of Technology) and worked at NASA/JPL and Boeing.
► Twitter: / rossdynamicslab
► Other Related Videos
▶️ Applications to Dynamical Astronomy • Interplanetary Transpo...
► Related Courses and Series Playlists by Dr. Ross
📚3-Body Problem Orbital Dynamics Course
is.gd/3BodyPro...
📚Space Manifolds
is.gd/SpaceMan...
📚Space Vehicle Dynamics
is.gd/SpaceVeh...
📚Lagrangian and 3D Rigid Body Dynamics
is.gd/Analytic...
📚Nonlinear Dynamics and Chaos
is.gd/Nonlinea...
📚Hamiltonian Dynamics
is.gd/Advanced...
📚Center Manifolds, Normal Forms, and Bifurcations
is.gd/CenterMa...
#orbitalmechanics #LagrangePoint #rotatingframe #threebodyproblem #CR3BP #space #3body #3bodyproblem #mathematics #SpaceManifolds #JamesWebb #NonlinearDynamics #gravity #SpaceTravel #SpaceManifold #DynamicalSystems #JamesWebbSpaceTelescope #space #solarSystem #NASA #dynamics #celestial #SpaceSuperhighway #InterplanetarySuperhighway #spaceHighway #spaceHighway #gravitational #mathematics #dynamicalAstronomy #astronomy #wormhole #physics #chaos #unstable #PeriodicOrbits #SaddlePoint #CriticalPoint #Halo #HaloOrbit #LibrationPoint #LagrangianPoint #LowEnergy #VirginiaTech #Caltech #JPL #LyapunovOrbit #CelestialMechanics #HamiltonianDynamics #planets #moons #multibody #GatewayStation #LunarGateway #L1gateway #ArchesOfChaos #cislunar #cislunarspace #orbitalDynamics #orbitalMechanics #celestialChaos #Chaotician #Boeing #JetPropulsionLab #Centaurs #Asteroids #Comets #TrojanAsteroid #Jupiter #JupiterFamily #JupiterFamilyComets #Hildas #quasiHildas #KuiperBelt

[@lucavisconti1872]

Hello, thanks for the video!
at 3:09 you say that the angular rate is "1". I believe that is the non-dimensional angular rate and it comes from how we have defined the non dimensional time: tao=nt (that is the angle of rotation of the m1-m2 line with respect the inertial x axis). So the non-dimensional angular rate is the derivative of tao with respect to tao, that is indeed 1. can you confirm?

[@ProfessorRoss]

Thank YOU for watching! I talk about the non-dimensionalization in the previous lecture (czcams.com/video/-j6Fv_Pb5fk/video.htmlsi=HttV5TXL-S4KTWBz&t=1511). But briefly, yes, we choose τ (which equals nt) as the unit of time, so the period of the m1-m2 system about its center of mass with respect to an inertial frame is 2π.

[@BruJacksonS2]

Hello, professor! Your classes are fascinating, thank you so much! However, I have a question: I have done a modeling using the normalized equations in the synodic system, centered on the barycenter. I already have the results I need because I found the initial conditions related to the orbits I was looking for. But I need to calculate the deltaV between the velocity of the initial condition in the synodic system that I found and the velocity of the spacecraft in the Earth's orbit corresponding to that altitude. Since the synodic system is centered on the barycenter and not on Earth, I'm confused about how to do this. I also need this information when the spacecraft is passing near the Moon (how much delta velocity would be needed to slow down the spacecraft and place it in a parking orbit). Remembering that the formula for a spacecraft to maintain a circular orbit around a body is given by sqrt(G*m/(R+H)), where R is the radius of the body (Earth or Moon) and H is the altitude of the spacecraft. OBS: I need this trough newtonian equations, not in lagrange or hamilton equations as you show in the next lectures.

[@ProfessorRoss]

You can do a transformation from the rotating (i.e., synodic) frame coordinates & velocity (x,y,z,v_x,v_y,v_z) & time t to an inertial frame centered on either the Earth's center or the Moon's center. For instance, if you are using the NON-DIMENSIONAL coordinates and time, then in the rotating frame, Earth is located at (d,0,0), where d=-mu. The transformation to the Earth-centered inertial frame coordinates and velocity (X,Y,Z,V_X,V_Y,V_Z) is:
X = c*(x-d) - s*y;
Y = s*(x-d) + c*y;
Z = z;
V_X = -s*(x-d + v_y) + c*(v_x - y);
V_Y = c*(x-d + v_y) + d*(v_x - y);
V_Z = v_z;
where c=cos(t), s=sin(t) and t is the nondimensional time. If you want Moon-centered inertial frame, use d=1-mu

[@stefanonovelli9353]

Prof.Ross ,Stefano Novelli: I'm following 3BP part 2:everything ok up until:csi prime,etaprime,zprime =Atau times xprime minus y,
yprime plus x ,z prime.The next step:Can you tell me? Thank you so much