
- 166
- 682 151
Jonathan Evans
Registrace 6. 09. 2010
My maths videos: some recreational ones involving pigs, some to provide background and extension material for my courses.
Time dilation
Thirteen years ago, I started to make a series of videos featuring Gizmo the Pig and Poob. The goal was to explain some of the weird effects of special relativity in a way that you could understand with a minimum of background. I made two of these videos: one czcams.com/video/_Z-1dkntITo/video.htmlfeature=shared about Pythagoras's theorem (all the background you really need) and one czcams.com/video/LGODWLbJeEE/video.htmlfeature=shared about how we know that light-speed is finite. I never got around to making any more. In this third video, our intrepid heroes finally come face to face with the sanity-sapping madness of time dilation. If the message of the video is lost on you, just remember that: Poob is always right.
zhlédnutí: 296
Video
Determinants and volume, revisited
zhlédnutí 877Před 2 lety
In this optional video for MATH105 (Linear Algebra) we give an alternative proof that the determinant of a matrix measures how volumes scale under the corresponding linear transformation.
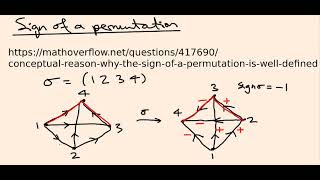
Sign of a permutation
zhlédnutí 1,1KPřed 2 lety
In this optional video for MATH105 (Linear Algebra) we prove that the sign of a permutation is well-defined. This fact was used when we defined determinants. The proof we give is inspired by this MathOverflow thread: mathoverflow.net/questions/417690/conceptual-reason-why-the-sign-of-a-permutation-is-well-defined/
Lie groups and Lie algebras: Invariant theory (optional extra video)
zhlédnutí 2,1KPřed 2 lety
We discuss in detail how to view the space of binary quadratic forms as a representation of SL(2,C) and why b^2 - 4ac is an invariant. This relies on the earlier video jde27.uk/lgla/28_plethysm.html and corrects a mistake in an earlier version of that video.
Lie groups and Lie algebras: Decomposing into irreps
zhlédnutí 2,2KPřed 2 lety
We do some worked examples of decomposing SU(2) representations into irreducible summands, including C^2 tensor C^2 and Sym^2 Sym^2 C^2. Note: This is a minor tweak of an earlier video to correct a mistake at the end (can you spot the not-so-subtle video editing?).
Lie groups and Lie algebras: Decomposing SU(3) representations
zhlédnutí 2KPřed 2 lety
We do a worked example in which we decompose the tensor cube of the standard representation of SU(3) into irreducible summands. Note: This is just a rerecording of an earlier video to fix a minor but confusing typo.
Lie groups and Lie algebras: Classification of SU(3) representations
zhlédnutí 3,4KPřed 2 lety
We describe the weight diagrams of irreducible SU(3) representations (proof will come in a later video). Note: This is just a re-recording of an earlier video where I messed up the conventions for \Gamma_{k,l}.
Lie groups and Lie algebras: Further reading
zhlédnutí 3,8KPřed 3 lety
A couple of book recommendations for further reading about Lie groups, Lie algebras and related topics. Very biased towards the books I know and like.
Lie groups and Lie algebras: Classification of Dynkin diagrams
zhlédnutí 2,7KPřed 3 lety
We sketch how you would approach the classification of Dynkin diagrams of compact semisimple Lie groups (the details are left as an in-depth project for students taking the course).
Lie groups and Lie algebras: Simple roots
zhlédnutí 1,9KPřed 3 lety
We define simple roots in a root system, describe how they define a Weyl chamber, and prove some properties of the simple roots. In particular, we prove that they form a basis of R^n.
Lie groups and Lie algebras: Classification of root systems, 2
zhlédnutí 1,9KPřed 3 lety
We introduce Dynkin diagrams and state the classification theorem.
Lie groups and Lie algebras: Dihedral angles
zhlédnutí 736Před 3 lety
We show that the angles between two mirrors (root hyperplanes) in a root system can only be 90, 60, 45 or 30 degrees.
Lie groups and Lie algebras: Classification of root systems, 1
zhlédnutí 1,9KPřed 3 lety
We start to discuss the classification of root systems.
Lie groups and Lie algebras: Root systems
zhlédnutí 3,9KPřed 3 lety
We introduce the notion of a root system, which abstracts the properties common to root diagrams of compact semisimple Lie groups. We prove that root diagrams have all the required properties.
Lie groups and Lie algebras: Root spaces are 1-dimensional
zhlédnutí 1,4KPřed 3 lety
We show that root spaces (for representations of compact semisimple groups) are 1-dimensional.
Lie groups and Lie algebras: sl(2,C) subalgebras in general, 2
zhlédnutí 966Před 3 lety
Lie groups and Lie algebras: sl(2,C) subalgebras in general, 2
Lie groups and Lie algebras: sl(2,C) subalgebras in general, 1
zhlédnutí 1,5KPřed 3 lety
Lie groups and Lie algebras: sl(2,C) subalgebras in general, 1
Lie groups and Lie algebras: Dual Killing form and semisimplicity
zhlédnutí 1,5KPřed 3 lety
Lie groups and Lie algebras: Dual Killing form and semisimplicity
Lie groups and Lie algebras: The Killing form
zhlédnutí 3,4KPřed 3 lety
Lie groups and Lie algebras: The Killing form
Lie groups and Lie algebras: The weight lattice
zhlédnutí 1,6KPřed 3 lety
Lie groups and Lie algebras: The weight lattice
Lie groups and Lie algebras Optional Extra: Any torus is contained in a maximal torus
zhlédnutí 749Před 3 lety
Lie groups and Lie algebras Optional Extra: Any torus is contained in a maximal torus
Lie groups and Lie algebras Optional Extra: Compact connected abelian groups are tori
zhlédnutí 819Před 3 lety
Lie groups and Lie algebras Optional Extra: Compact connected abelian groups are tori
Lie groups and Lie algebras Optional Extra: Finding compact abelian subgroups
zhlédnutí 934Před 3 lety
Lie groups and Lie algebras Optional Extra: Finding compact abelian subgroups
Lie groups and Lie algebras: Maximal tori, overview
zhlédnutí 1,4KPřed 3 lety
Lie groups and Lie algebras: Maximal tori, overview
Lie groups and Lie algebras Optional Extra: Uniqueness of irreps with given highest weight
zhlédnutí 638Před 3 lety
Lie groups and Lie algebras Optional Extra: Uniqueness of irreps with given highest weight
Lie groups and Lie algebras Optional Extra: Proof of classification of SU(3) irreps
zhlédnutí 962Před 3 lety
Lie groups and Lie algebras Optional Extra: Proof of classification of SU(3) irreps
Lie groups and Lie algebras: The quark model
zhlédnutí 2,6KPřed 3 lety
Lie groups and Lie algebras: The quark model
Lie groups and Lie algebras: Weyl symmetry
zhlédnutí 2,2KPřed 3 lety
Lie groups and Lie algebras: Weyl symmetry
Lie groups and Lie algebras: Root vectors acting on weight spaces
zhlédnutí 2,7KPřed 3 lety
Lie groups and Lie algebras: Root vectors acting on weight spaces



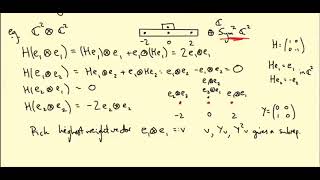




this helped me so much! cannot thank you enough!!!
Lie groups are dual to lie algebras. Vectors (contravariant) are dual to co-vectors (covariant) -- Dual bases. Riemann curvature is dual, upper indices are dual to lower indices, contravariant is dual to covariant. Injective is dual to surjective synthesizes bijection or isomorphism. "Always two there are" --Yoda. Sine is dual to cosine or dual sine -- the word co means mutual and implies duality.
This is amazing, thank you so much! (context: trying to draw a ellipsis from a covariance matrix)
Subgroups are dual to subfields -- the Galois correspondence. Reducible is dual to irreducible -- non reducible is dual to non irreducible! Null homotopic is dual to non null homotopic. Symmetry (waves, Bosons) is dual to anti-symmetry (particles, Fermions) -- quantum duality. "Always two there are" -- Yoda. "The negation of the negation synthesizes the positive" -- Hegel. Positive is dual to negative -- numbers or electric charge.
Injective is dual to surjective synthesizes bijective or isomorphism (duality). Antipodal points identify for the rotation group SO(3) -- north poles are dual to south poles (magnets). "Always two there are" -- Yoda. Spinors -- you have to travel around a mobius loop twice to get back to your original position.
5:10
Convergence (syntropy) is dual to divergence (entropy). Teleological physics (syntropy) is dual to non teleological physics (entropy). Ellipsoids are dual to hyperboloids. "Always two there are" -- Yoda.
8:37 I'm not 100% sure but I think it might be that iλ∈(i Lie(T))* since λ:Lie(T)->ℝ but I might be being overly pedantic by pointing that out.
Not enough schools teach students the fact the "complex plane" is really a real algebra for a bivector (plus scalar). Bivectors (unit bivectors, or more precisely unit rotors) generate rotations in _any_ space dimension whatsoever. Every bivecotr plane defines a complex structure, henceforward geometer should really just talk about "bivector structure" which is more linguistically meaningful, and ditch the term "complex". Scalars are ... well... dilations, of course. So the branch cuts and Riemann surfaces obtained thereby have these periodicities because they are an obscure way to encode rotational symmetry (also Lorentz boosts for a Lorentzian bivector/rotor). More complicated functions could be thought of as more generalized bivector transformations, the Riemann curvature tensor, for example, is best thought of as a bivector valued function of a bivector and a spacetime position. All Lie groups are spin groups (generated by bivectors). geometry.mrao.cam.ac.uk/1993/01/lie-groups-as-spin-groups/
This great material suffers hugely from volume being way too low. I have to jack up the computer volume to the max just to make out barely what you're saying, and as long as there's no ambient noise in my area.
Well done Jonathan. Thanks
14:46 I think ℂ^3 should be something like (ℂ^3)^ ⊗^3
@15:00 I don't really get how you comput the derivative of the left side of this equation, can you elaborate on that?
He just reuses what he computed few line before.
15:20: I was having great difficulty showing that any complex line was a ℂ subrepresentation of the irreducible representation of ℂ^n. I did some digging and found Schur’s Lemma. I found that I was able to show that this was a subrepresentation R|ℂ=π1∘R∘ι Where π1:ℂ^n->ℂ was the projection and ι:ℂ->ℂ^n was the embedding. Then f:ℂ->ℂ^n defined by f(z)=ι(mz) was the U(1)-linear map required to show isomorphism by Schur’s Lemma since R∘f=f∘R|ℂ, this would prove that ℂ=ℂ^n, hence n=1. So I found it is not so much that any complex line is a sub-rep, it is more so that scaling the number and taken with embedding and projection would make a sub-rep and prove isomorphism by Schur’s Lemma. (Unless I missed something or am overcomplicating things).
Thank you very much for this video and for the whole playlist, which I have enjoyed a lot! I liked that you ended the series by providing examples. In particular, you addressed the point I raised in a comment to some earlier video about the existence of a universal cover. The universal cover of the figure 8 is exactly the example I tried to describe in that comment. I had wrongly named it “free space” (basically, I was looking at it as a free tree construction, if that makes sense). It’s kind of funny because the figure 8 is what corresponds to the free group on two generators. While its universal cover corresponds to the trivial group (which could also be seen as a free group, I guess, but without generators). Back to the main point. At the end of the video, you hinted at the fact that there are spaces that don’t admit a universal cover. I thought about several options (using variants of the topologist’s sine curve, one of the weirdest spaces I know). But so far I haven’t been able to find a good counter-example. I will do some research then. Thanks again!
Are there any path-connected, simply path-connected spaces, which do not admit a simply connected universal cover? I may be wrong, but it seems to me like any loop can be unfolded to get some “free space” (I put it in quotes, cause I’m not sure if the term is accurate - but, hopefully, it should be understandable). For instance, take the figure 8. The middle point is a cross. So, it can be unfolded into an infinite tree, each node of which has 4 edges, connecting it to 4 other nodes. The covering map would then map every node in this infinite tree to the single node of the figure 8. Two of the four incident edges would be mapped to one loop (but going in opposite directions), while the other two to the other loop (again, each one following one direction of travel). It’s not hard to see that this space is simply connected and the map is a covering map. Of course, it’s just one example. But it seems to have some degree of generality, which is why it makes me wonder whether the requirement that X admits a universal cover is needed or not..
Let me see if I understand covering isomorphisms. As far as I can tell, the difference between a homeomorphism and a covering isomorphism lies in the presence of the covering maps. So, whenever I have a homeomorphism F : X_1 -> X_2 and a covering map p_1 : X_1 -> Y, I can make a new covering map p_2 = p_1 \circ F^{-1} from X_2 to Y, such that F : (X_1, p_1) -> (X_2, p_2) is a covering iso between them. Moreover, given p_1 and F, p_2 is uniquely defined as above, as follows from the definition of covering transformation. However, a different iso F’ would give rise to a different covering map p_2’. I believe this reasoning should be correct.
Thanks again for this video (I love your content, as you can probably tell). I have two questions: 1) In the first theorem, shouldn’t we also assume that Y_1 is path-connected and locally path-connected? I guess it may be implied by the fact that p_1 is itself a covering map (so that any path in the base space can be lifted to a path in the covering space and this should be enough, somehow). But shouldn’t we justify it? 2) As the video shows, a covering transformation “decomposes” a covering map as the composition of two covering maps (possibly under certain conditions, see comment above). Can we also build covering maps by composing covering maps? This seems trivially true to me. I believe it should follow from the definition of covering map (and we shouldn’t even need to impose any constraints on any of the spaces). But I might be wrong, of course.
Still thinking about (1) above. Looking more carefully at the definition of covering map/covering space: www.homepages.ucl.ac.uk/~ucahjde/tg/html/cov-01.html en.wikipedia.org/wiki/Covering_space they don’t seem to imply that the covering space should be path-connected (or even connected). It may even contain points which are outside of the union of all the sheets. Now, we can always restrict our attention to the open subset given by the union of the elementary sheets, and then apply the lifting result once for each connected component of that space. But, to ensure uniqueness of the lifting, we would need to pick a point in each connected component (since the fundamental group is about loops based at a point). We would also need to require local path-connectedness, to ensure continuity. Any thoughts?
I think I said something stupid about the covering space containing points, which lie outside of the union of the elementary sheets. This could not be the case, since a covering map is a (total) function, first of all. So, Y_1 is definitely covered by the elementary sheets of p_1. But, that aside, I think that my point about requiring Y_1 to be both path-connected and locally path-connected should still be valid.
I’ve just realized that you left that condition out on purpose (pre-class question #2): www.homepages.ucl.ac.uk/~ucahjde/tg/html/gal-02.html You scared me there! ;)
I’m not sure I understand why we need to use the inclusion condition on the push-forwards to prove well-definedness of the lifting. Couldn’t we just lift the loop from X to Y? Wouldn’t the two halves of the lifted loop be themselves liftings of the two corresponding halves of the X loop (by definition of path lifting - along with path composition)? This would imply, in particular, that the inclusion condition is true of every path-connected, locally path-connected T, covering map p : Y -> X and continuous function f : T -> X. What am I missing?
Ok, I think I see why. Loops in T may be homotopically equivalent to the constant loop (this was not an issue in the case of path lifting or homotopy lifting, because the test spaces, [0,1] and [0,1]x[0,1] resp., were simply connected). As a result, it may not be possible to find a single elementary neighborhood which includes the pushforward of such a loop in its totality. Therefore, the lifting of such a loop to the covering space wouldn’t be a loop at all, making f tilde not well-defined. For instance, if T=S^1 and X is the torus, with f mapping T around the torus (either direction, it doesn’t matter). Then the lifting of (the pushforward of) a non-trivial loop in S_1 would be homotopically equivalent to a straight line in R^2. In particular, the starting and ending points would not be idetified, hence the ambiguity.
As always, thanks for the cool video! In the proof that the quotient map is a covering map, we are using the group G to index the copies of the quotient space. So, we are implicitly endowing it with the discrete topology. In the cases of G equal Z or Z/n, that’s just its natural topology. Are there any non-trivial examples of properly discontinuous (continuous) group actions, where the group in question isn’t normally given the discrete topology (e.g. where G=R)?
I guess we can always choose X to be R x {0,1}, so that Y = {0,1} and R plays the role of the group/index set. The group action could be a translation by a real (keeping the ‘0’/‘1’ label constant). I don’t know if this would be of any interest though. Probably, "non-trivial" wasn’t the best word to describe what I was after. I meant “interesting”, although that can be subjective. Maybe a slightly more interesting example could be X=C (the complex numbers) and G=R/(2\pi), with G acting on X by way of rotations. So that Y = X/G = [0,\infty). Just thinking out loud, I haven’t checked the details...
As I said, I was thinking out loud. Neither of the above examples satisfy proper discontinuity.
Perhaps the second example I gave above (X=C and G=R/(2\pi)) does work, provided we assign a somewhat unusual topology to C. Namely, let the topology be generated by all the open sets of the non-negative reals (open sets of radii) at each angle (real number in the [0, 2\pi) interval). For example, one such open set could be: U_\theta = \{ re^{i\theta} | r\in U \} for some fixed angle \theta and U\subseteq [0, \infty). Note that this topology is finer than the standard one on C. I’m not sure if it already has a name, but I’m pretty sure somebody must have thought about it before. So, with this topology on C, we can let G act on it by rotating points, and this should satisfy the proper discontinuity condition. Another similar example would be to keep the real part fixed and let R act on C by vertical translations in imaginary space. Of course, there’s nothing special about the vertical direction, we can translate in any direction we want. In each of these cases, the required topology on C would be a small variation on the one I wrote above (they are all homeomorphic). Sorry for the rambling. It helps me to clarify my thoughts (and, hopefully, might stimulate others too).
The big face reveal! Nice face.
The volume is too soft. Hard to hear you
😂😂😂😂😂😂😅😅😅😅😅😅😅😅😅
Thanks for providing notes along with each video! Just to spell it out (and please correct me if I’m wrong), the two elementary nbhds that cover the figure 8 are the figure 8 without the cross point and an "open cross" around that point (whose pre-image would be two open crosses around the two cross points). In particular, we can’t have two branches of the cross joined, or we would lose continuity of the map h: p^{-1}(U) -> U x {0,1} (where U is the open cross). You ask: “Are there any more 2-to-1 covers of the figure 8?”. How about, two disconnected figure 8’s?
I love this stuff, thanks for the video! I have a couple of questions about the relations between generators, which I recap here for convenience (trying my best not to add any errors): 1) \sigma_i \sigma_j = \sigma_j \sigma_i (when | j - i | >= 2) 2) \sigma_i \sigma_{i+1} \sigma_i = \sigma_{i+1} \sigma_i \sigma_{i+1} And that’s fine. My question is about the case i=j. Initially, I was tempted to think that \sigma_i would be its own inverse, since applying \sigma_i twice would simply swap the same two points twice. But it can’t be, cause the group elements are the (homotopy classes of) paths in (unordered) configuration space. So, if you make the i-th point go behind the (i+1)-th and then do the same thing again, the result is the two points going around each other in a loop. But that can’t be equivalent to the identity (two straight strands), can it? Am I correct to assume that \sigma_i^{-1} would consist of making the (i+1)-th point go behind the i-th point instead? (i.e. right strand goes behind the left, which is the opposite of what the \sigma_i’s do in your video)
That’s crazy! I’m thinking about the implications for orientable surfaces with genus g>1. And I’m sure there are even weirder examples that can be drastically simplified using Van Kampen's theorem. Beautiful!
I was a little puzzled by the definition of mapping torus. Due to the presence of the [0,1] interval, I immediately thought of it as a homotopy from id_X to \phi, even it never says that in the video. And, indeed, it doesn’t have to be. In particular, it isn’t necessarily continuous (when seen as a deformation of id_x into \phi along [0,1]). I guess what fooled me was the fact that \phi itself is continuous. The example of the Klein bottle is what made me confront my misconception. I’m just putting it out there, just in case.. and please correct me if I’m wrong.
Thanks again for another great video! I’m wondering if the definition of HEP could be simplified by letting X=Y, F=id_X and A x [0,1] -h-> A (where Y on the rhs became A). It seems to be what you’re actually using in this particular proof and it would require one quantifier less (for all F …). I’m not sure if it would be equivalent to your definition in general though. In other words, is every homotopy of type A x [0,1] -h-> B always decomposable into a homotopy of type A x [0,1] -h-> A, followed by some A -f-> B ?
Ok, it wasn’t too hard to check that the answer to my question is "no, they’re not equivalent”. It suffices to let A be the singleton set and B=[0,1] , with h isomorphic to id_B. Then A x [0,1] -h'-> A can only be the constant map, and there is no A -f-> B such that h = f\circ h’. Then the true definition of HEP (the one you gave) is stronger than my “simplified variant”. But I’m still wondering if there are any interesting cases that mine is too weak to capture.
I would like to see an example of a quotient map which is not open
In the video, after he states the theorem, he says that it doesn't apply to the example, because [0, 2\pi) is not compact. But, since F is a bijection, I was tempted to check if its inverse satisfied the conditions. After all, S^1 seems compact to me. Similarly, [0, 2\pi) seems to meet the criteria for Hausdorffness… However, it is continuity that fails in this case, I believe. In particular, [0, \theta) \subset [0, 2\pi) is open, but it's pre-image isn’t. Any thoughts?
Please reply Sir, which is the reference book
Thank you for the beautiful videos! I think that the formula may not be correct. At t=(1-s)/2, the argument of alpha should be 0 (for all s). However, the 2t part cancels with s-1 and the -st is generally negative (except when s=0 or s=1).
This one seems to work: [ t - (1-s)/2 ] / [ 1 - (1-s)/2 ], which is equal to (2t - 1 + s)/(1+s). Now: when t = (1-s)/2, the above is 0; whereas, when t=1, the expression is also 1.
Good
Does this help with number theory?!! How can it be used?! For example, if I wanted to prove Fermat's Last theorem, how could it be used?
There are jumps which aren't clear for me (and possibly for the audience). I really thought we were going to talk about the fact that if we shrink the fast winding large loop into the point, then it must, at some R, intersect zero. And the fact that a constant polynomial different from zero would always result in a point P(x) different from zero. I really don't see where homotopy is used here? Anyone can explain? Thanks.
31:19 This is not in the video, but in the written version of the presentation found on the professor's website. Here he wrote: this implies that uv is in the normal subgroup generated by the amalgamated relations. I don't quite understand how one reaches this conclusion, especially when we are talking about u_1v_1...u_nv_n. Anybody could help, please?
Aren’t u the one who “owned” Shapiro?
Quit screwing around and get to your damn point. Your presentation deserves a thumbs down.
very nice video :) youre very good at explaining, thank u!
If Poob has a million fans, then I am one of them. If Poob has ten fans, then I am one of them. If Poob has only one fan then that is me. If Poob has no fans, then that means I am no longer on earth. If the world is against Poob, then I am against the world.
Thank you for making these lectures public - they're great to learn from. I got a different interpolation - alpha( (2/(1+s)) t - (2/(1+s) -1)), hopefully doesn't matter as long as there is at least one homotopy then they're equivalent, right?
Thank you
thanks for the video. 9:54 det(..) = t^2-t-1
@quentinmerrit loserrrrrr
Just finished this playlist!❤ Thanks
Thank you, I've been struggling to understand this in my lecture notes (y1 math) 👍 This made the summation part clearer :DD
(u,v)should be written as u+v
why killing form can be viewed as an inner product? Normally, y needs to be transposed tr(xy^T) to make it positive definite.
May I ask what tool you are using to make these videos? Thank you.
hope you can add representation of sp(2n) and its application on mechanics.